Speed of Light: Case History

Everyday experiences suggest that although sound travels fast, its speed can be determined without much trouble. We stand a distance of ten miles from a nuclear explosion and hear the sound of that explosion some 48 seconds after we have seen it. From this we can conclude that sounds takes 48 seconds to travel 10 miles, or one fifth of a mile (1,086 feet) in one second. Similarly, when we see someone hitting a log of wood at a distance and hear the sound of split wood a fraction of a second later, we can obtain a rough estimate of the speed of sound.

When we come to light, everyday experiences fail us, for they strongly suggest that light takes no time at all to travel from one point to another. Imagine two observers standing 2 miles and 200 miles, respectively, from a nuclear explosion. Imagine further that they have nothing better to do at that time than precisely clock the time they first see the explosion. As far as the best clocks on Earth can tell, they would see the light this explosion yields at the exact same time. Similarly, when I smile at myself in the mirror, the image I see is produced by light bouncing from my face to the mirror and back. As far as I can tell, at the same instant I am conscious of having produced a smile, I see myself smiling. All this of course raises a very interesting question: Does light take any time to travel from one place to another, or does it traverse distances in no time at all? Is light simply faster than sound, or does it possess infinite speed? Does the light produced by far away galaxies take time to reach us, or does it reach us the moment it is given off?

By now we know well the answer to this question, but this is a fairly recent development in the history of ideas. Most of the ancient Greeks, including the ubiquitous Aristotle, believed that the velocity of light was infinite; that is, that light would take 0 seconds to travel from one end of the universe to the other. Others disagreed. Empedocles (490-435 B.C.), for instance, believed that the speed of light was finite; that light took a certain amount of time to travel from one point to another.

An interesting argument in favor of the proposition that light takes no time to travel from one point to another was provided by Hero of Alexandria. Today we believe that sight involves reflection of light from an object and its absorption by the eye. But Hero, like most Greeks, believed that we see because the eye produces light. Now, Hero said, if I look skywards on a starry night, close my eyes for a while, then open them, I see the very distant stars instantly. It therefore must take the light from my eyes no time at all to travel to the faraway stars. Therefore, light travels at an infinite speed.

The subject remained controversial for millennia. In the early eleventh century, two noted Arab scientists, Avicena and Alhazen, provided commonsense arguments in favor of the proposition that the velocity of light is finite. Alhazen, for instance, felt that light involves a movement of something, and therefore that it cannot be at two places at the same time. The Englishmen Roger Bacon (1212-1292) and Francis Bacon (1561-1626) also felt that light traveled with a finite speed.

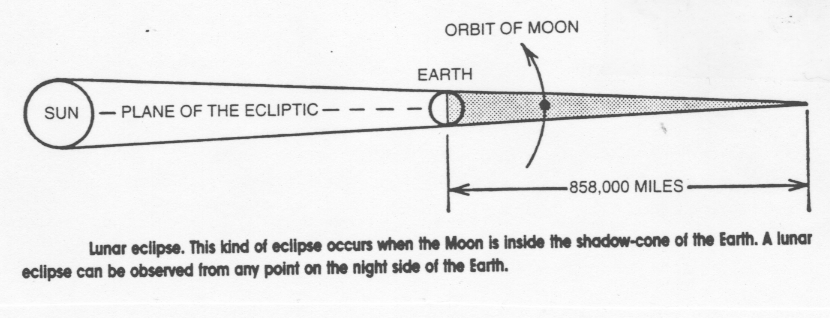
By the time of the great astronomer and physicist Johannes Kepler's (1571-1630), the issue still remained unresolved. Kepler subscribed to the view that light emitted at point A would be seen instantly at point B, even if the distance between the 2 points was billions of miles. Rene Descartes (day-KHART; 1596-1650), the great philosopher, mathematician, and scientist, not only shared Kepler's views, but was the first to provide an excellent argument in favor of the proposition that light took no time to travel from one point to another. Consider, Descartes said, a lunar eclipse. The Moon, Descartes knew, does not shine by its own light; what we call moonlight is simply a reflection of sunlight. Occasionally, Sun, Earth, and Moon form a straight line, with the Earth between the two. The Moon then is in Earth's shadow, and cannot be seen. We call this event a lunar eclipse.

The Moon, Descartes reasoned, is extremely far from Earth. So, if light does not reach instantaneously from one point to another, it must take the Moon's reflected light some finite time to reach us. Let's say light would take only one hour to travel the immense distance from the Moon to Earth.

Now, Descartes continued, let us consider a lunar eclipse. The Moon has just entered the shadow of the Earth, so that Sun, Earth, and Moon form a straight line. At this point, Earth blocks sunlight from reaching the Moon. But the sunlight that had already passed the Earth will continue traveling towards the Moon for another hour. It will then be reflected back to us and take another hour to reach us. We shall therefore become aware of the eclipse two hours after the Sun, Earth, and Moon formed a straight line. By then, the Moon will be farther along in its orbit around the Earth. In other words, if light takes an hour to travel from Earth to the Moon, at the time we see the eclipse, the Sun, Earth, and Moon will no longer form a straight line. But this is contrary to astronomical observations: when we see the eclipse, Sun, Earth, and Moon do form an exact line. Therefore, concluded Descartes, light takes no time to travel from one point to another.

An even more interesting idea for resolving this question was proposed by Galileo (gahl-ih-LAY-oh; 1564-1642) and carried out many years later by members of the Florentine Academy. This experiment involved two investigators standing at, say, two hilltops one mile apart. Let's imagine that these two guys are actually Galileo and his friend. The night is clear and dark. Both observers hold covered lanterns. Galileo uncovers a lantern and simultaneously clocks the time. As soon as his friend sees the light from Galileo's lantern, he uncovers his lantern. When Galileo sees the light from his friend's lantern, he records the time (as best he can; the clock was not yet invented). The experiment is repeated many times and the combined times averaged. The observers then repeat the same procedure at a distance of ten miles. Now, the only difference between the two sets of observations is the distance between Galileo and his friend. If light travels at a finite speed, it would take longer to travel one mile and back than 10 miles and back. Yet, no difference was detected, suggesting again that light either takes no time to travel from one point to another, or that it does it too fast to be measured from a distance of a few miles.

By 1665 another famous scientist, Robert Hooke, noted that Descartes' and Galileo's experiments failed to prove the instant propagation of light. What they did prove, Hooke felt, was, that if light took time to travel from one point to another, it did so "exceeding quick." If, for instance, light took two minutes instead of two hours to go past Earth to the Moon and back to Earth, the deviation from straight line of Sun, Earth, and Moon during an eclipse would be too small to be detected. A similar argument could be raised against Galileo. If light took a very small fraction of a second to travel 10 miles, its motion would appear instantaneous. In other words, Galileo's and Descartes' observations were inconsistent with a comparatively slow speed of light, but they still left open the question whether light took unimaginably short time to travel great distances or whether it took no time at all to travel any distance.

By 1676, the question whether light takes time to travel from one point to another, or whether it takes no time at all had been resolved by the Danish astronomer, Ole Roemer. Roemer provided the first clearcut proof that light takes time to move from one point to another, and the first reasonable estimate of its actual speed.

Jupiter's moons.gif (53937 bytes)

Selected moons of Jupiter, drawn to scale. Starting from top: Io, Europa, Ganymede, and Callisto (courtesy: NASA)

To the naked eye, the planet Jupiter appears as a single, steady, point of light. Jupiter was one of the first objects to attract Galileo's attention when he directed his telescope skywards. To his astonishment, Jupiter no longer appeared as a single point of light, but as a small disk, surrounded by four moons in orbit about it.

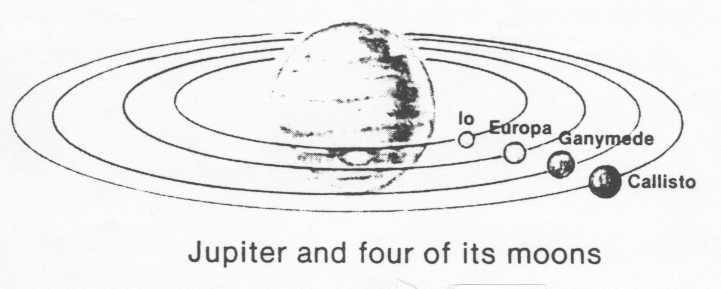In the decades following Galileo's discovery, these moons received a great deal of attention from astronomers. Astronomers knew by then that an object in space takes a fixed time to complete one revolution about another object. Earth, for instance, takes one year to complete one revolution about the Sun. The Moon, similarly, takes 27.3 days to complete one revolution about Earth. It would appear reasonable therefore to suppose that Io, for instance, completed one revolution about Jupiter in a fixed period of time.

But how do you calculate how long it takes Io to travel about Jupiter exactly once? Galileo himself noticed that, even on cloudless nights, Io disappeared from sight at given intervals. It seemed to get closer and closer to Jupiter, and then to vanish behind it. Sometime later it would reappear on the other side of Jupiter. The most reasonable interpretation for this was an eclipse. At a certain portion of its orbit about Jupiter, Io cannot be seen by Earthly observers because it is behind Jupiter. As it continues in its orbit, it reappears on the other side.

This gave astronomers the tool they needed to determine the revolution of Io around Jupiter. They measured the exact time Io gets lost from view and the time it gets lost from view again. The difference between the two is the time it takes Io to complete one revolution about Jupiter. To allow for experimental error, you repeat the measurements a few times and average the results out. The mean, culled from many years' observations by Roemer and others, turned out to be 42 hours, 28 minutes, and 34 seconds.

But in this case, statistics and calculations of averages provide a misleading picture, for the time intervals between two successive eclipses varied. It was precisely these unexpected variations that led Roemer to his discovery of the speed of light.

To understand Roemer's basic procedure, it will be more convenient to ignore the real numbers he worked with, and to arbitrarily assume that Io takes exactly 2 days to complete one revolution about Jupiter. Let us say that at point 3 in the figure below, the beginning of Io's eclipse has been recorded at exactly 11:08 p.m. January 3, 1672. Six days later we observe another eclipse at point 4 at 11:09 p.m. What could be the possible explanation for this additional minute? By January 9, the Earth moved a considerable distance from Io. Light from Io would have to travel the same distance it traveled before, plus the distance between points 3 and 4, before it could be perceived by an Earthly observer. After six more days pass, the eclipse would be seen at 11:10, and so on, until, when the Earth reached the farthest point in its orbit away point from Io, the eclipse would be seen at 11:16. On the way back, the reverse process would occur, with the eclipse recorded on Earth first at 11:16; six days later, 11:15; at point 5, 11:09, at point 3, 11:08; all the way back to something like 11:00 when the Earth comes closest in its orbit to Io.

Roemer of course had to work with an average interval of 42 hours, 28 minutes, and 34 seconds for one eclipse, so his calculations were more cumbersome than the ones above, but the idea behind them was the same. He now knew how long it took light to go from one side of Earth's orbit to the other, and he knew the distance itself (twice the distance of the Earth from the Sun). If you know the distance an object travels in space, and the time it takes this object to travel this distance, you can calculate this object's velocity. For instance, a car that covers 500 miles in 5 hours travels at an average speed of 500/5 = 100 miles per hour.

Roemer observations told him that when Earth was farthest away from Jupiter, eclipses of Io were observed on Earth some 16 minutes later than when Earth was closest in its orbit to Jupiter. So, he concluded, light takes 16 minutes to cover the diameter of Earth's orbit. Since the diameter was known, he was able to come up with 140,000 miles per second as the speed of light. Today we have a more accurate figure for the speed of light (186,000 miles in one second), but this does not detract in any way from Roemer's achievement. His observations and deductions showed, for the first time, that light takes time to travel from one point to another. They also gave a fairly close approximation of the actual speed of light and showed that light was unimaginably fast. Roemer was also able to explain Galileo's and Descartes' failures. At a distance of a few miles, light travels too fast to be measured. It would, for instance, take a mere 1/18,600 of a second for light to travel 10 miles. Even moonlight reaches Earth in just 1.3 seconds.

The first independent confirmation of Roemer's discovery was given by James Bradley in 1729, nineteen years after Roemer's death. Bradley was also able to arrive at a much more accurate estimate. Others have used a variety of ingenious approaches and instruments to further refine this value, so that now we are reasonably certain that light travels some 186,000 miles in one second, and use this value repeatedly in both astronomical and physical calculations.

It is perhaps worth noting that Roemer's observations were repeated in 1790 and again in 1874. With the better instruments available then, the maximum lag time observed for Io's eclipse was 16 minutes and 34 seconds. Combined with the more accurate estimates of the diameter of the earth's orbit (186,000,000 miles) then available, these more accurate data yield 186,000,000 miles / 994 seconds, or 187,000 miles per second for the speed of light. This fairly close estimate shows that Roemer's approach made sense, and that it fell short by 46,000 miles per second for technical reasons.

Roemer's discovery of the speed of light has played a decisive role in the history of physics and astronomy. For instance, in one of the best known equations of physics (E=mc²), c stands for the speed of light. This equation says that, if you convert a given mass into energy, you can calculate the amount of energy you get by multiplying the mass by 186,000 and again by 186,000. Or roughly, to find out the quantity of energy, you need to multiply the mass by 35 billion! This is why, in nuclear explosions, a little bit of uranium or hydrogen produces enormous amounts of energy.

Today, we have excellent reasons to believe that nothing in the universe can possibly travel faster than 186,000 miles in one second. Nothing, that is, can go faster than the speed of light. As things stand now, we can observe the stars, but, because they are light years away, we cannot visit them. As Isaac Asimov put it: " The scientists . . . who tried to measure the speed light, little knew they were measuring the prisons bars that may keep us in the solar system forever."

Source: Moti Nissani. Permission for the free use of this material is hereby granted.